Find the Taylor series expansion of this expression By default, taylor uses an absolute order, which is the truncation order of the computed series T = taylor (1/ (exp (x)) exp (x) 2*x, x, 'Order', 5) T = x^3/3 Find the Taylor series expansion with a relative truncation order by using OrderModeGiven that x 3 4y 3 9z 3 = 18xyz and x 2y 3z = 0 x 2y = 3z, 2y 3z = x and 3z x = 2y Now `( x 2y )^2/(xy) (2y 3z)^2/(yz) (3x x)^2/(zxNumber of terms is equal to nr−1C n For (xy z)n r =3 Hence number of terms are n3−1C n = n2C n

Product Share In Customer Wallet Case Study Xyz Bank Information Pdf Powerpoint Templates
(x+y)^3 expansion
(x+y)^3 expansion-It can never be $3^n$ As you can see for $(ab)^n$ contains just $n1$ terms Note that we have to keep the sum of powers in each of the combinations of $x,y,z$ to $n$, so it will be reduced Now replace $a$ and $b$ by $x$ and $(yz)$ respectively So total number of terms should be $123\cdots(n1)=\dfrac{(n1)(n2)}{2}$You can use f(x,y) for the function if you prefer, but that's one more letter to keep track of




Q3hawsaplicwim
If the coefficients of x 3 and x 4 in the expansion of ( 3 k x) 9 are equal, then the value of k is KEAM 16 5 The coefficient of x 4 in the expansion of ( 1 − 2 x) 5 is equal to KEAM 17 6 If the 7 t h and 8 t h term of the binomial expansion ( 2 a − 3 b) n are equal, then 2 a 3 b 2 a − 3Consider the expansion of (x y z) 10 In the expansion, each term has different powers of x, y, and z and the sum of these powers is always 10 One of the terms is λx 2 y 3 z 5 Now, the coefficient of this term is equal to the number of ways 2x′s, 3y′s, and 5z′s are arranged, ie, 10!Obtain The Taylor Expansion Of Z(x, Y) Near (0,0) Upto The Second Order Using The Gradient And Hessian Matrix Z(x, Y) Is Given In Implicit Form As F(x, Y, Z) = Z Cos X X2 – Eyz = 0 This problem has been solved!
Share It On Facebook Twitter Email 1 Answer 1 vote answered by Juhy (631k points) selected by Vikash Kumar Best answerX 0 0 = 3 {substituted 0 for y and z} x = 3 {combined like terms} coordinates are (3, 0, 0) Algebra 3 Section 35 Systems with Three Variables The graph of an equation in three variables, such as, Ax By Cz = D where A,B, and C are not all zero, is a plane yintercept x y z = 3 {the equation} 0 y 0 = 3 {substituted 0 for x and zAnswer by lenny460 (1073) ( Show Source ) You can put this solution on YOUR website!
Binomial Expansions Binomial Expansions Notice that (x y) 0 = 1 (x y) 2 = x 2 2xy y 2 (x y) 3 = x 3 3x 3 y 3xy 2 y 3 (x y) 4 = x 4 4x 3 y 6x 2 y 2 4xy 3 y 4 Notice that the powers are descending in x and ascending in yAlthough FOILing is one way to solve these problems, there is a much easier wayBinomial Expansion Calculator is a handy tool that calculates the Binomial Expansion of (xyz)^10 & displays the result ie, x^10 10x^9y 10x^9z 45x^8y^2 90x^8yz 45x^8z^2 1x^7y^3 360x^7y^2z 360x^7yz^2 1x^7z^3 210x^6y^4 840x^6y^3z 1260x^6y^2z^2 840x^6yz^3 210x^6z^4 252x^5y^5 1260x^5y^4z 25x^5y^3z^2 25x^5y^2z^3 1260x^5yz^4The number of distinct terms in the expansion of (x y – z)16 is (a) 136 (b) 153 (c) 16 (d) 17 binomial theorem;




The Number Of Terms In The Expansion Of X Y Z 10 Is
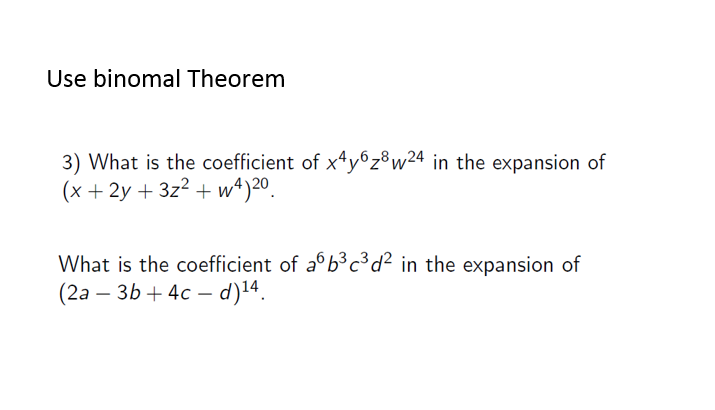



Use Binomal Theorem 3 What Is The Coefficient Of Chegg Com
SOLUTION Since the number of terms in the expansion of (xyz) n is (n1) (n2)/2 The number of terms in the expansion of (x y z) 10 is (101) (102)/2=11X12/2 =11X65!) Thus, (xyz) 10 = ∑(10!) / (P1About Press Copyright Contact us Creators Advertise Developers Terms Privacy Policy & Safety How works Test new features Press Copyright Contact us Creators




Sample Exam 1 Solution Digital Logic Spring 03 Ece 240 Docsity



Digoo Sb Xyz Wireless Bluetooth And Wifi Smart Home Hd Video Doorbell Mullally S Electronics
Compute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, science, nutrition, history (xy)^3 (yz)3 (zx)^3 = 3(xy)(yz)(zx) That is it no constraints etc It mentions "This can be done by expanding out the brackets, but there is a more elegant solution" Homework Equations The Attempt at a Solution First of all this only seems to hold in special cases as I have substituted random values for x,y and z and they do not agreeThe Taylor expansion is the standard technique used to obtain a linear or a quadratic approximation of a function of one variable Recall that the Taylor expansion of a continuous function f(x) is The value of ∂u(x i, y i)/




Formula Of X Y Z 3 Novocom Top



Example 15 If X Y Z Are Different Show 1 Xyz 0 Class 12
Therefore, F = m3 m4 m5 m6 m7, which is the same as above when we used term expansion x y z Minterms Notation 0 0 0 x' y' z' m0 0 0 1 x' y' z m1 0 1 0 x' y z' m2 0 1 1 x' y z m3 1 0 0 x y' z' m4 1 0 1 x y' z m5 1 1 0 x y z' m6 1 1 1 x y z m7 Table 39 F = x' y z x y' z x y z' x y zThis is the Solution of Question From RD SHARMA book of CLASS 9 CHAPTER POLYNOMIALS This Question is also available in R S AGGARWAL book of CLASS 9 You can F The number of terms in the expansion of (xyz) n Related questions 0 votes 1 answer If the integers r > 1, n > 2 and coefficients of (3r)th and (r 2)nd terms in the binomial expansion of (1 x)2n are equal, then asked in Class XI Maths by rahul152



Example 15 If X Y Z Are Different Show 1 Xyz 0 Class 12
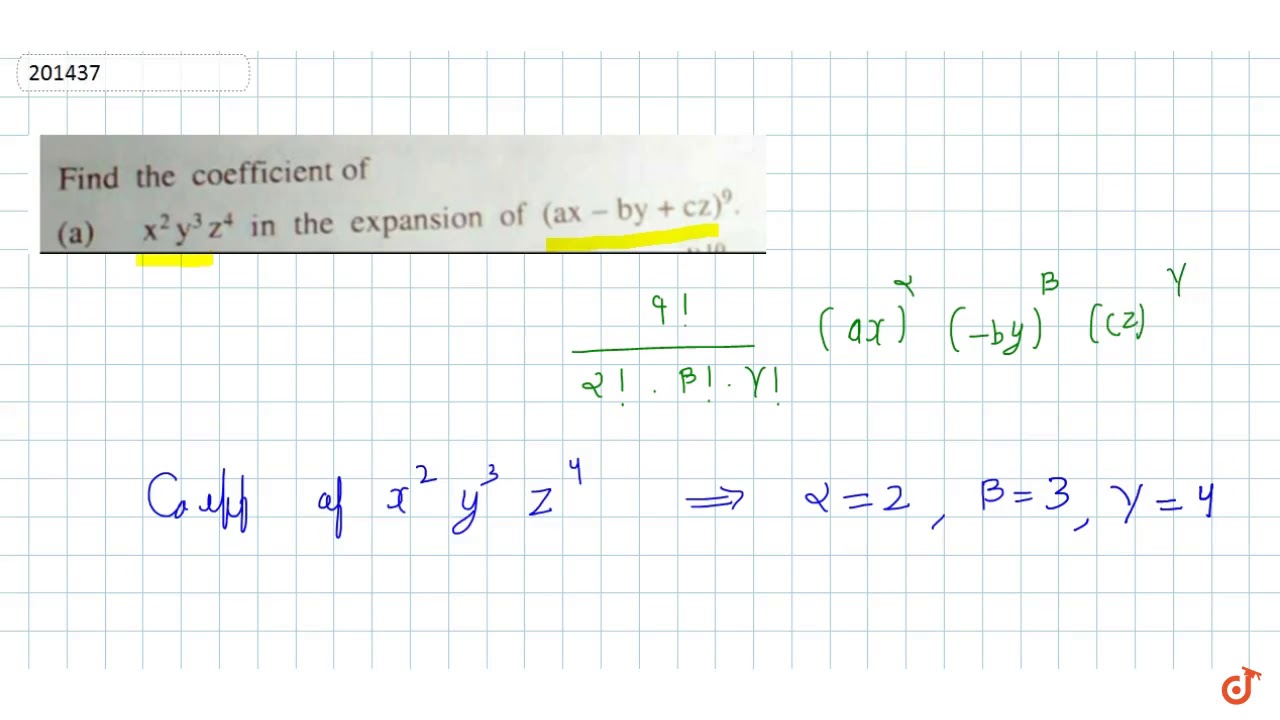



Find The Coefficient Of X 2 Y 3 Z 4 In The Expansion Of Ax By Cz 9 Youtube
Adding 3abc both side a^3 b^3 c^3 = (a b c) (a^2 b^2 c^2 ab ac bc) 3abc Now, put a=x, b=y, and c=z x^3 y^3 z^3 = (x y z) (x^2 y^2 z^2 xy xz yz) 3xyz Put xyz =0 (Given) x^3 y^3 z^3 = (0) (x^2 y^2 z^2 xy xz yz) 3xyz x^3 y^3 z^3) rr Adopt the standard subscriptcomponent conventions x' = x 1 ', y' = x 2 ' and z' = x 3 'Question from Binomial Theorem,cbse,math,class11,ch8,binomialtheorem,exemplar,q26,secb,medium




Xyz Wikipedia
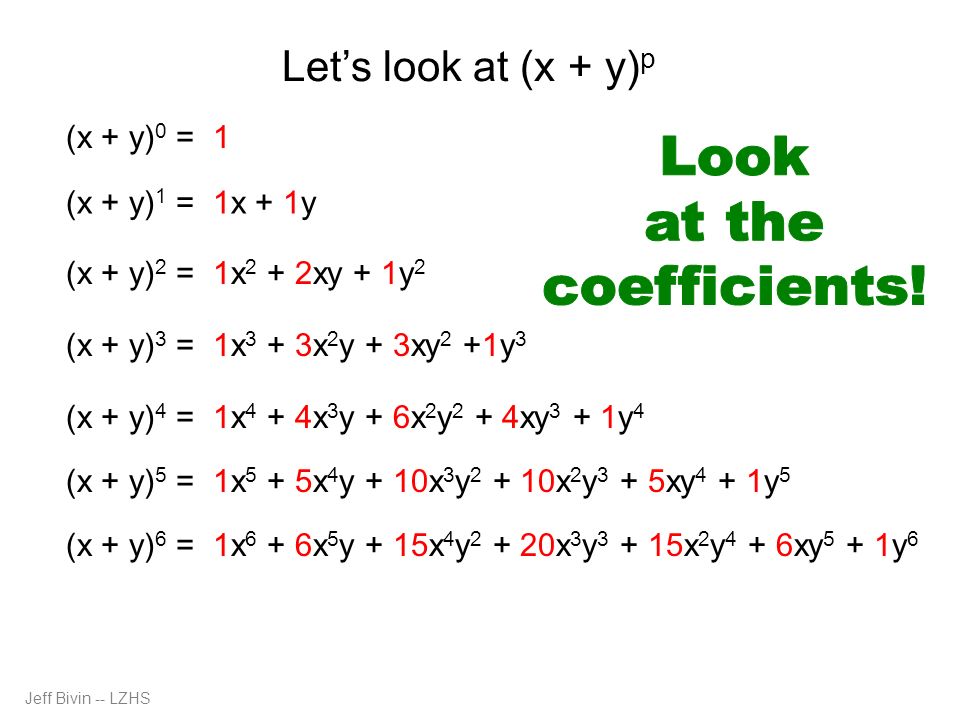



Binomial Expansion And More Ppt Download



0 件のコメント:
コメントを投稿